Nucleoids, Janus Particles, and Active Matter

Topics

Flow through the Nucleoid

Janus Particle Propulsion

Active Particle Simulations

Flow through the Nucleoid

Hard Inner Spherocylinder

Soft Potential

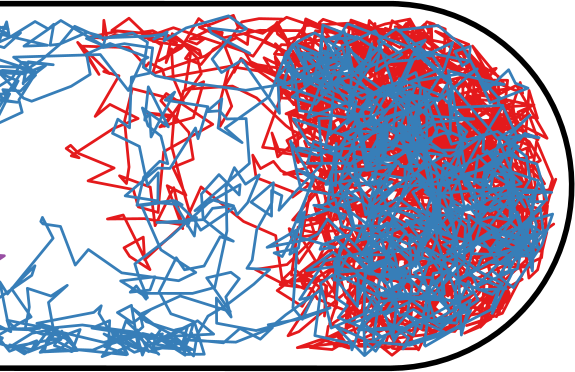
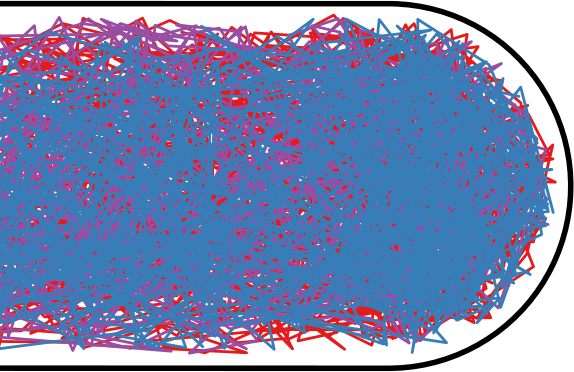
Flow through the Nucleoid

Wiggins

Soft Potential

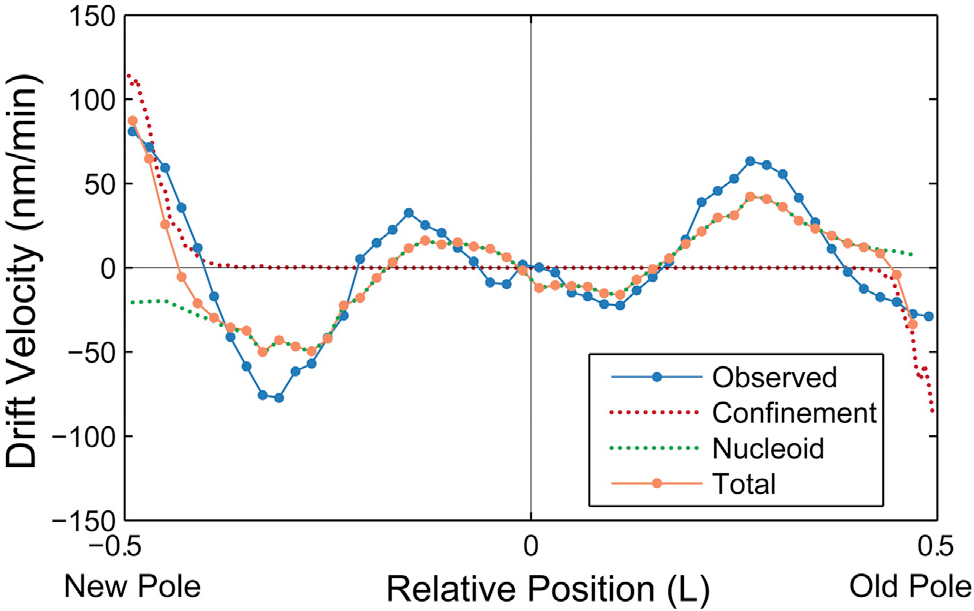
Passive Model

If we combine the predicted drift velocity maps for the nucleoid exclusion and membrane confinement models, we see striking agreement with the qualitative shape and quantitative scale of the observed drift velocity map throughout the entire cell.

— Stylianidou, Kuwada, and Wiggins [3]

They use an entirely passive model, like ours, to predict the same nucleoid flow

Position Dependent Diffusion Constant

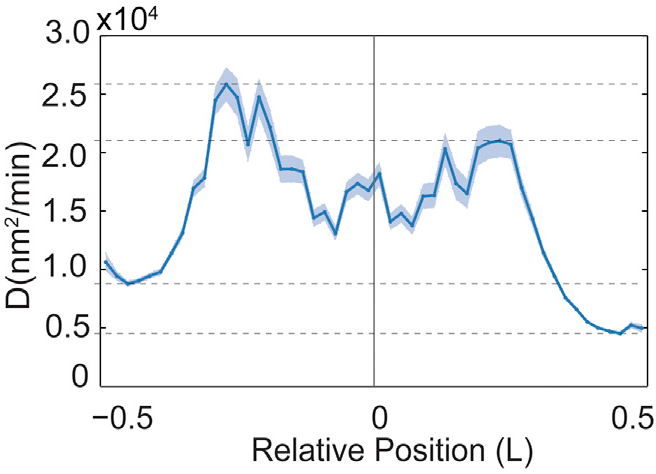
Fig. 4B from Wiggins

“The nucleoid-exclusion model would naively predict both decreased occupancy over the nucleoid as well as a decreased diffusion coefficient. Strikingly, our results show exactly the opposite phenomenology: comparison between the occupancy of MS2-mRNA (Fig. 2 A) and the position dependent diffusion coefficient (Fig. 4 B) reveals that the highest diffusion coefficients are observed in regions with the lowest MS2-mRNA occupancy and the highest nucleoid density.”

Position Dependent Diffusion Constant

Fig. 4B from Wiggins

Soft Potential

Diffusion constant calculated from

\[ D \sim \frac{\left< \Delta x^2 \right>}{\Delta t} \]

Step Size Distribution

Wiggins

Soft Potential

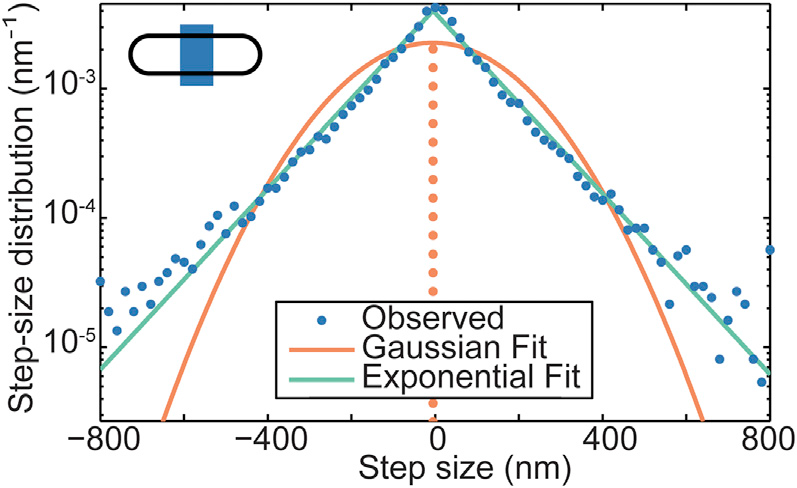
Janus Particle Propulsion

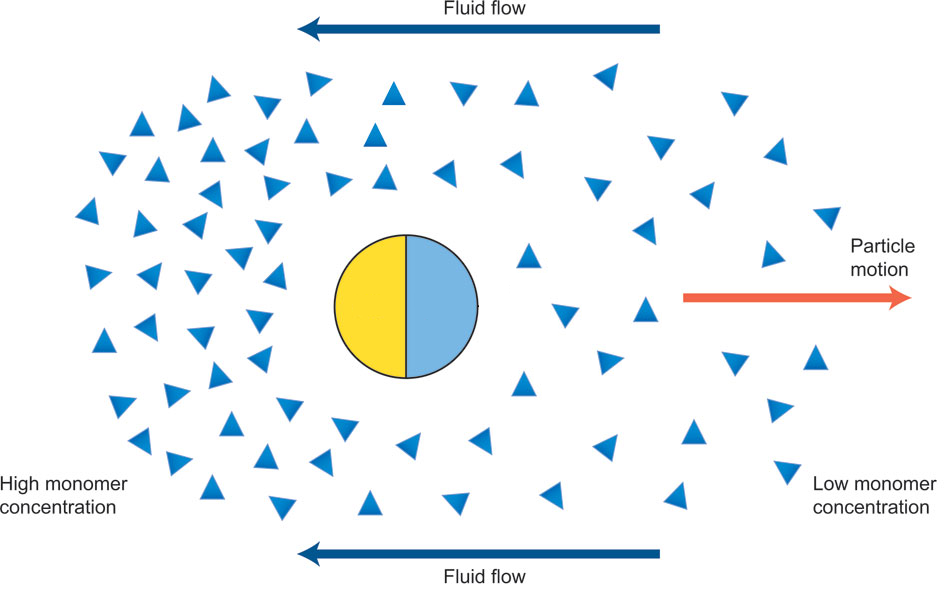
Half of each particle is coated with platinum, which catalyzes \(2 \mathrm{H_2 O_2 \rightarrow 2 H_2 O + O_2}\) on only one side
“The particles are being propelled by the local osmotic pressure gradient created by the asymmetric chemical reaction.” [2]

Note	Image is a modified diagram from a different paper [1].

Janus Particles

Janus Particle Trajectories in varying concentrations of H₂O₂

Janus Particles

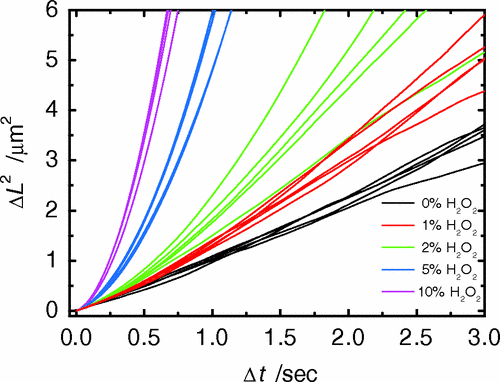
Particles in H₂O₂ move much farther

Active Particle Simulations

Metabolic activity does not significantly raise the temperature of the cell (right?)
ATP, the main energy source of metabolism, has energy \(E_\mathrm{ATP} \sim 20 k_B T\)
Metabolic activity would not have a rotational orientation
Individual events happen infrequently, relative to the diffusion coefficients

Simulating Metabolism

Langevin thermostat \[ \vec F = -\vec \nabla U - \gamma \vec v + \vec \Gamma_T + \vec \Gamma_k\left(t\right) \]
- WCA potential / repulsive Lennard-Jones for \(U\)
- Damping \(\gamma\) to simulate solution viscosity
- Random, instantaneous "kicks" to the particles
  - \(\vec \Gamma_T\) for the thermostat; balanced by \(\gamma\), the drag force
  - \(\vec \Gamma_k\) for metabolism

Random Kicks

Thermostat	Metabolism
\(\Gamma_T \sim \Delta t k_B T \sim {10}^{-5} k_B T\) Every timestep Mimic "bumping into water molecules" Correlated: satisfy Fluctuation-Dissipation Theorem Gaussian distribution Balanced by the drag force \(-\gamma \vec v\)	\(0 \le E_k \le 20 k_B T\) Instantaneous Infrequent, ~100—1000 timesteps Uncorrelated Uniform distribution

Thermostat

Metabolism

\(\Gamma_T \sim \Delta t k_B T \sim {10}^{-5} k_B T\)
Every timestep
Mimic "bumping into water molecules"
- Correlated: satisfy Fluctuation-Dissipation Theorem
- Gaussian distribution
- Balanced by the drag force \(-\gamma \vec v\)

\(0 \le E_k \le 20 k_B T\)
Instantaneous
Infrequent, ~100—1000 timesteps
Uncorrelated
Uniform distribution

Simulation

Without Activity

With Activity

Experimental MSDs

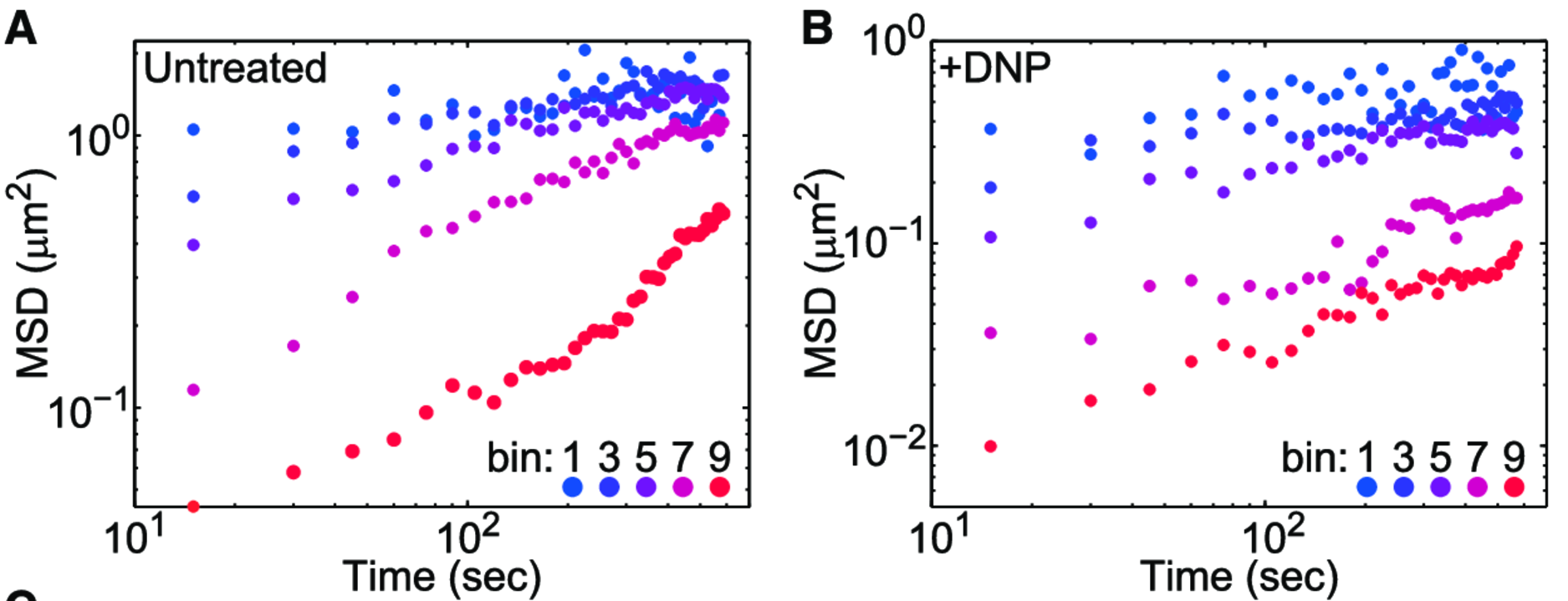
A factor of \(\sim 2-10 \)

MSDs

with \(20 k_B T\) Kicks

MSDs

with \(200 k_B T\) Kicks

MSD Comparison

Bibliography

B. Feringa, Nat Chem 3, 915 (2011).
J. R. Howse, R. A. L. Jones, A. J. Ryan, T. Gough, R. Vafabakhsh, and R. Golestanian, Phys. Rev. Lett. 99, 048102 (2007).
S. Stylianidou, N. J. Kuwada, and P. A. Wiggins, Biophysical Journal 107, 2684 (2014).